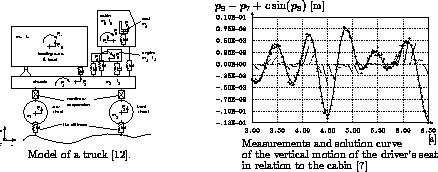
Figure 2: An example of parameter identification in vehicle dynamics.
The dynamic behavior of robots or vehicles can be described as multibody systems (after an index reduction) by
.
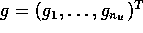For a sufficiently accurate simulation of the real system
the knowledge of specific data is required as, e.g.,
for robots the moments of inertia and
friction parameters,
and for vehicles the damping coefficients.
Within a controlled experiment functions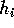 
, 
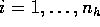, of the state variables
(and also the input functions of the system) are measured at
discrete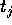 
, 
,
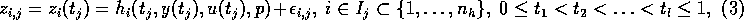
where 
is the (unknown) measurement error.
The task is to estimate p in such a way that the experiment that is simulated
by a numerical integration of (2) does optimally fit the measurements.
As a criterion for optimality
the weighted nonlinear least squares objective
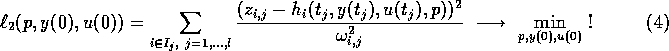
with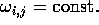 
can be used, where 
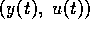is the solution of (2) for
the parameters p and the initial values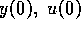 
that might be unknown, too.
